Fourier-transform Ion Cyclotron Resonance Mass Spectrometry (FT-ICR-MS)
Fourier-transform ion cyclotron resonance mass spectrometry
(FT-ICR-MS) is often thought of as being the most complex method of
mass analysis and detection. When considered at first principles
though, it is fairly simple to describe - although this does ignore
many of the 3 dimensional ion perturbation effects which make the
technique far more complex. It is not necessary to discuss those in
any detail here. The technique of ICR-MS was first published in the
mid. 1950's [1] where it was demonstrated for measurement of very
small mass differences at very high precision. The technique remained
a largely academic tool until the application of FT methods [2] by
Alan Marshall and Melvin Comisarow in the early 1970's [3]. It is now
one of the most sensitive methods of ion detection in existence and
has almost unlimited resolution - >107 is possible, with
most experiments taking place in the 105 to 106
range.
In the basic FT-MS instrument, the ions are generated in the source and pass through a series of pumping stages to increasingly high vacuum. When the ions enter the cell (ion trap) pressures are in the range of 10-10 to 10-11 mBar with temperatures close to absolute zero. The cell is located inside a spatial uniform static superconducting high field magnet (typically 4.7 to 13 Tesla) cooled by liquid helium and liquid nitrogen. When the ions pass into the magnetic field they are bent into a circular motion in a plane perpendicular to the field by the Lorentz Force (see figure 1 and equation 1). They are prevented from leaving the cell by the trapping plates at each end.
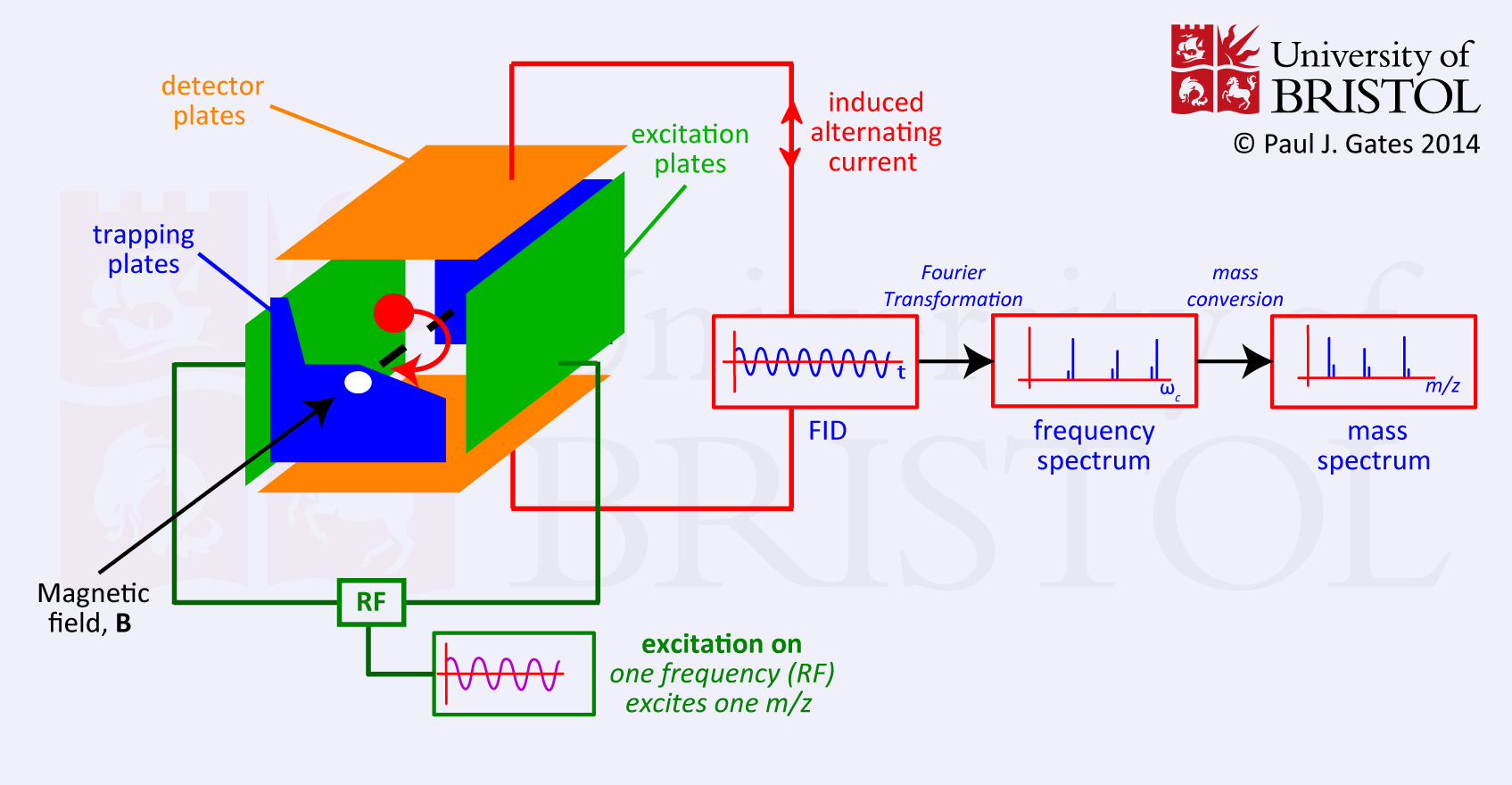
The frequency of rotation of the ions is dependent on their m/z ratio (equation 2). At this stage, no signal is observed because the radius of the motion is very small. Excitation of each individual m/z is achieved by a swept RF pulse across the excitation plates of the cell. Each individual excitation frequency will couple with the ions natural cyclotron motion and excite them to a higher orbit where they induce an alternating current between the detector plates. The frequency of this current is the same as the cyclotron frequency of the ions and the intensity is proportional to the number of ions. When the RF goes off resonance for that particular m/z value, the ions drop back down to their natural orbit (relax) and the next m/z packet is excited. Although the RF sweep is made up of a series of stepped frequencies, it can be considered as all frequencies simultaneously. This results in the measurement of all the ions in one go producing a complex frequency vs. time spectrum (the convoluted frequency spectrum or FID) containing all the signals. Deconvolution of this signal by FT methods results in the deconvoluted frequency vs. intensity spectrum which is then converted to the mass vs. intensity spectrum (the mass spectrum) by equation 3 (the mass conversion). It is also usual to correct for mass errors at this stage by applying a calibration.
Due to the ion-trap nature of FT-MS, it is possible to measure the ions without destroying them, this enables further experiments to performed on the ions. The most common of these would be some kind of fragmentation study (MS/MS or MSn) for structural elucidation experiments, but also other gas-phase reactions and studies can be performed - e.g. gas-phase basicity calculations, gas-phase kinetics, ion dissociation studies as well as the study of ion-molecule or ion-ion interactions. Alan Marshall has a published a number of reviews of FT-ICR and its applications over the years [4].
[3] M.B. Comisarow and A.G. Marshall, Chemical Physics Letters, 25, 1974, p282 and Journal of Chemical Physics, 62, 1975, p293 and Journal of Chemical Physics, 64, 1976, p110.
[4] A.G. Marshall, Accounts of Chemical Research, 18, 1985, p316 and Accounts of Chemical Research, 29, 1996, p308.
In the basic FT-MS instrument, the ions are generated in the source and pass through a series of pumping stages to increasingly high vacuum. When the ions enter the cell (ion trap) pressures are in the range of 10-10 to 10-11 mBar with temperatures close to absolute zero. The cell is located inside a spatial uniform static superconducting high field magnet (typically 4.7 to 13 Tesla) cooled by liquid helium and liquid nitrogen. When the ions pass into the magnetic field they are bent into a circular motion in a plane perpendicular to the field by the Lorentz Force (see figure 1 and equation 1). They are prevented from leaving the cell by the trapping plates at each end.

The frequency of rotation of the ions is dependent on their m/z ratio (equation 2). At this stage, no signal is observed because the radius of the motion is very small. Excitation of each individual m/z is achieved by a swept RF pulse across the excitation plates of the cell. Each individual excitation frequency will couple with the ions natural cyclotron motion and excite them to a higher orbit where they induce an alternating current between the detector plates. The frequency of this current is the same as the cyclotron frequency of the ions and the intensity is proportional to the number of ions. When the RF goes off resonance for that particular m/z value, the ions drop back down to their natural orbit (relax) and the next m/z packet is excited. Although the RF sweep is made up of a series of stepped frequencies, it can be considered as all frequencies simultaneously. This results in the measurement of all the ions in one go producing a complex frequency vs. time spectrum (the convoluted frequency spectrum or FID) containing all the signals. Deconvolution of this signal by FT methods results in the deconvoluted frequency vs. intensity spectrum which is then converted to the mass vs. intensity spectrum (the mass spectrum) by equation 3 (the mass conversion). It is also usual to correct for mass errors at this stage by applying a calibration.
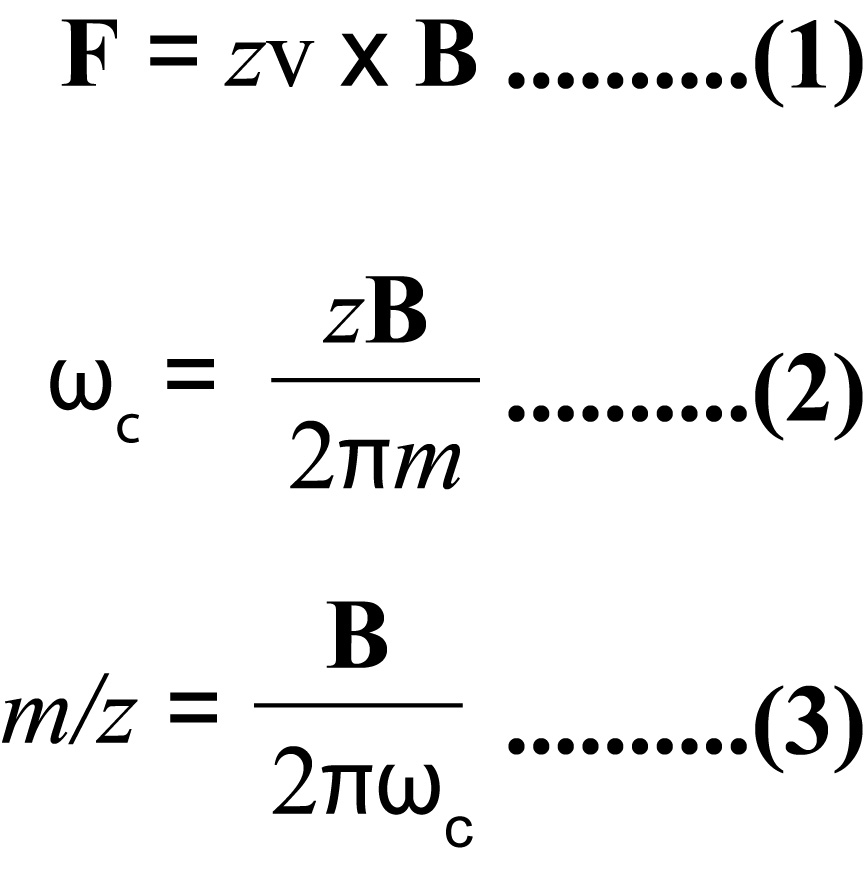 |
|
Due to the ion-trap nature of FT-MS, it is possible to measure the ions without destroying them, this enables further experiments to performed on the ions. The most common of these would be some kind of fragmentation study (MS/MS or MSn) for structural elucidation experiments, but also other gas-phase reactions and studies can be performed - e.g. gas-phase basicity calculations, gas-phase kinetics, ion dissociation studies as well as the study of ion-molecule or ion-ion interactions. Alan Marshall has a published a number of reviews of FT-ICR and its applications over the years [4].
References:
[1] J.A. Hipple et al., Physical Review, 76, 1949, p1877 and Physical Review, 82, 1951, p697.[3] M.B. Comisarow and A.G. Marshall, Chemical Physics Letters, 25, 1974, p282 and Journal of Chemical Physics, 62, 1975, p293 and Journal of Chemical Physics, 64, 1976, p110.
[4] A.G. Marshall, Accounts of Chemical Research, 18, 1985, p316 and Accounts of Chemical Research, 29, 1996, p308.