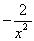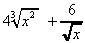
These are to be discussed in your tutorial in week 10.

1. Differentiate each of the following functions with respect to x:

(a) sin (4x) (b) cos (&pi-2x) (c) sin xcos x

(d) e^{sin x} (e) ln (cos x) (f) cot x

2. Each hydrogen atom in a hydrocarbon molecule in its lowest energy state oscillates at a frequency of 9x10¹³ s^-1 with an amplitude of ±10pm. The expression for the time dependence of the displacement of a hydrogen atom from its equilibrium position is x = x₀ sin (2t). Differentiate this with respect to time. Hence find its maximum velocity, and the maximum kinetic energy of vibration of a mole of such hydrogen atoms.

3. Integrate with respect to x

(a) 3x² (b) 4x⁵ -3x² +2 (c) 1 - 2x⁷

4.Integrate:

(a) 4t² (b) 8m³ -2 (c) 4Φ⁴ - &Phi (d) 120ξ⁷⁹ + ξ² (e) φ³+4φ -2

5. What is the area under the curves:

(a) y = 3x² + 1 between x = 1 and x = 4 ?

(b) p = 2q³ - 2 between q = 5 and q = 6

(c) λ = 5θ - θ² between θ = 0 and θ> = 1

6. Integrate the following with respect to x:

(a) 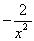(b) (c) 3x (d)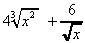

(e) e^6x (f) exp (-3x) (g) 4e^2x (h)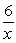

(i) 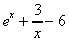(j) 3cos x (k) 2sin x - 3sec² x (l) sin 5x (m) 4cos 2x