Returning to the example of HCl from lecture one, at any fixed internuclear separation the best wavefunction is a mixture of two wavefunctions, y_ion and y_cov, describing respectively an ionic form of HCl (both electrons on the chlorine), and a covalent form (one electron on each atom).

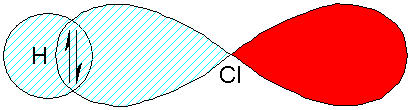Y_cov

H⁺ Cl^– Y_ion

Y = c_ion Y_ion + c_cov Y_cov

The coefficients c_ion and c_cov can be found by the variational method (see lecture 1).

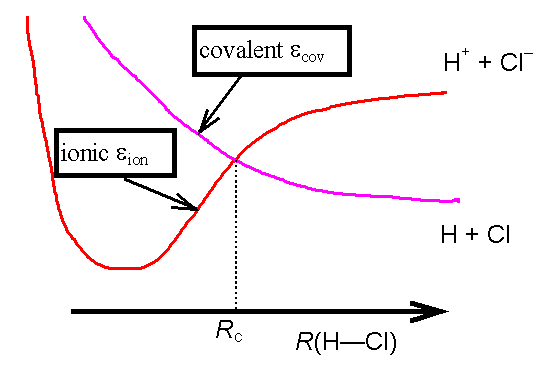
This is the situation at a particular fixed H--Cl internuclear separation. At different internuclear separations, the coefficients found by the variational method will be different. For example, HCl dissociates into neutral atoms, so y_cov (which has one electron in the Cl 3p orbital, and one in the H 1s orbital) is a better description at large internuclear separations. At short distances, though, the molecule is much more polar, with more tendency for both electrons to be associated with the chlorine, so y_ion is a better description. As the nuclear separation changes the potential energy curves corresponding to the “ionic” and “covalent” wavefunctions cross.

However, we get a better description (a better wavefunction) by allowing the ionic and covalent wavefunctions to mix, giving two new ‘mixed’ states with energy E₁ and E₂.

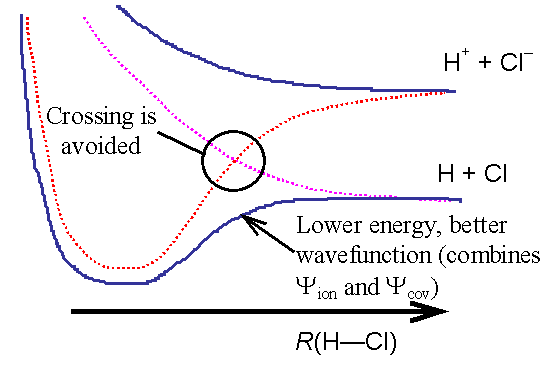
At every internuclear separation the original energies split apart. The new energies “E” are better in the sense that they are closer to the exact correct results. At points where the original energies (e_ion and e_cov ) are very close (or cross) the new better energies split apart and no longer cross. The point of closest approach is called an avoided crossing point.

More on the non-crossing rule