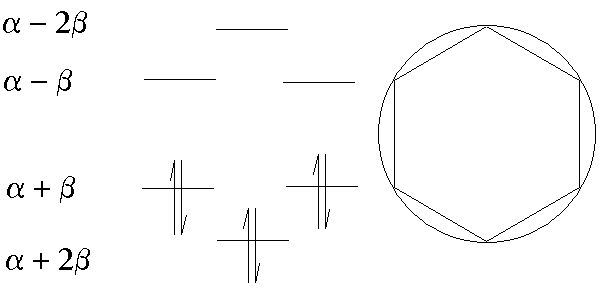The Circle Method
A geometric
representation of solutions of the secular equations for cyclic conjugated
polyenes
· A particularly
useful (and easy) way of finding the MOs of cyclic conjugated polyenes is the ‘Geometric’
or ‘Circle’ Method.
· In this method,
a diagram of the polyene is inscribed in a circle of radius 2b.
· One apex of
the polyene must be at the bottom.
· The vertical
axis represents energy.
· The centre of
the circle has an energy a.
· The lowest
point of the circle corresponds to an energy of (a + 2b). Note that b is negative, so this is a lower energy than a.
· There is a
molecular orbital at the energy corresponding to where the corners are
situated.
For example:
3-membered ring
(cyclopropenyl radical)

________________________________________
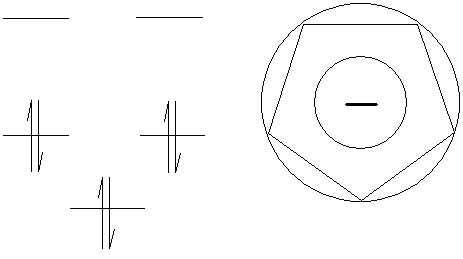
5-membered ring
(cyclopentadienyl anion)
________________________________________
6-membered ring
(benzene)